
Properties of Rational Functions and Expressions Video 2
Finding horizontal asymptotes for rational functions
Problem: Find the horizontal asymptotes for the following rational functions:

Finding horizontal asymptotes for rational functions

Determining the end behavior and horizontal asymptotes for rational functions

Properties of exponential growth and decay functions
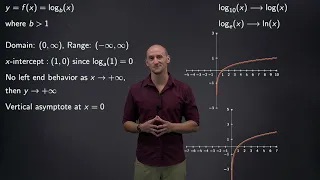
Explaining the basic properties of logarithmic functions
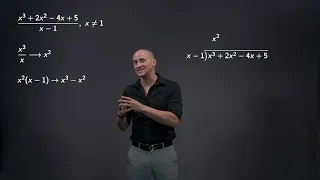
Explaining how to do polynomial long division
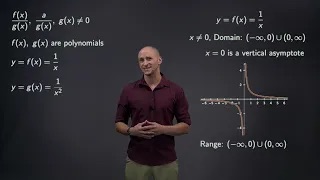
Discussing parent functions of rational functions and their properties

Determining the end behavior, real zeros, domain, and y-intercept for a polynomial

Determining the end behavior, real zeros, domain, and y-intercept for a polynomial

Determining the end behavior, real zeros, domain, and y-intercept for a polynomial

Determining the end behavior, real zeros, domain, and y-intercept for a polynomial

Determining the end behavior, real zeros, domain, and y-intercept for a polynomial

Finding the domain, intercepts, asymptotes, and holes of a rational function

Finding the domain, intercepts, asymptotes, and holes of a rational function

Finding the domain, intercepts, asymptotes, and holes of a rational function

Dividing rational expressions and simplifying

Subtracting rational expressions and simplifying

Performing operations with rational expressions and simplifying
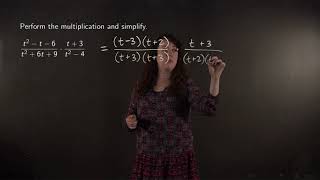
Performing operations with rational expressions and simplifying

Finding the end behavior of a polynomial

Finding the end behavior of a polynomial

Finding the end behavior of a polynomial

Finding properties of a polynomial including zeros and end behavior

Finding properties of a polynomial including zeros and end behavior

Finding properties of a polynomial including zeros and end behavior
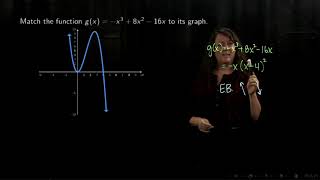
Using properties of a polynomial to find its graph
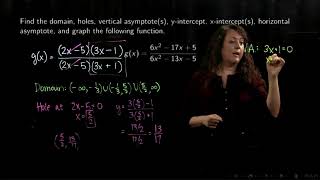
Determining the properties of a rational function and graphing it
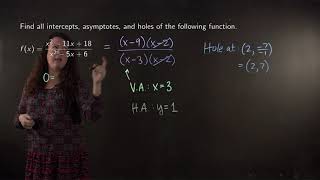
Determining the properties of a rational function and graphing it

Review of limits, continuity, and the Intermediate Value Theorem
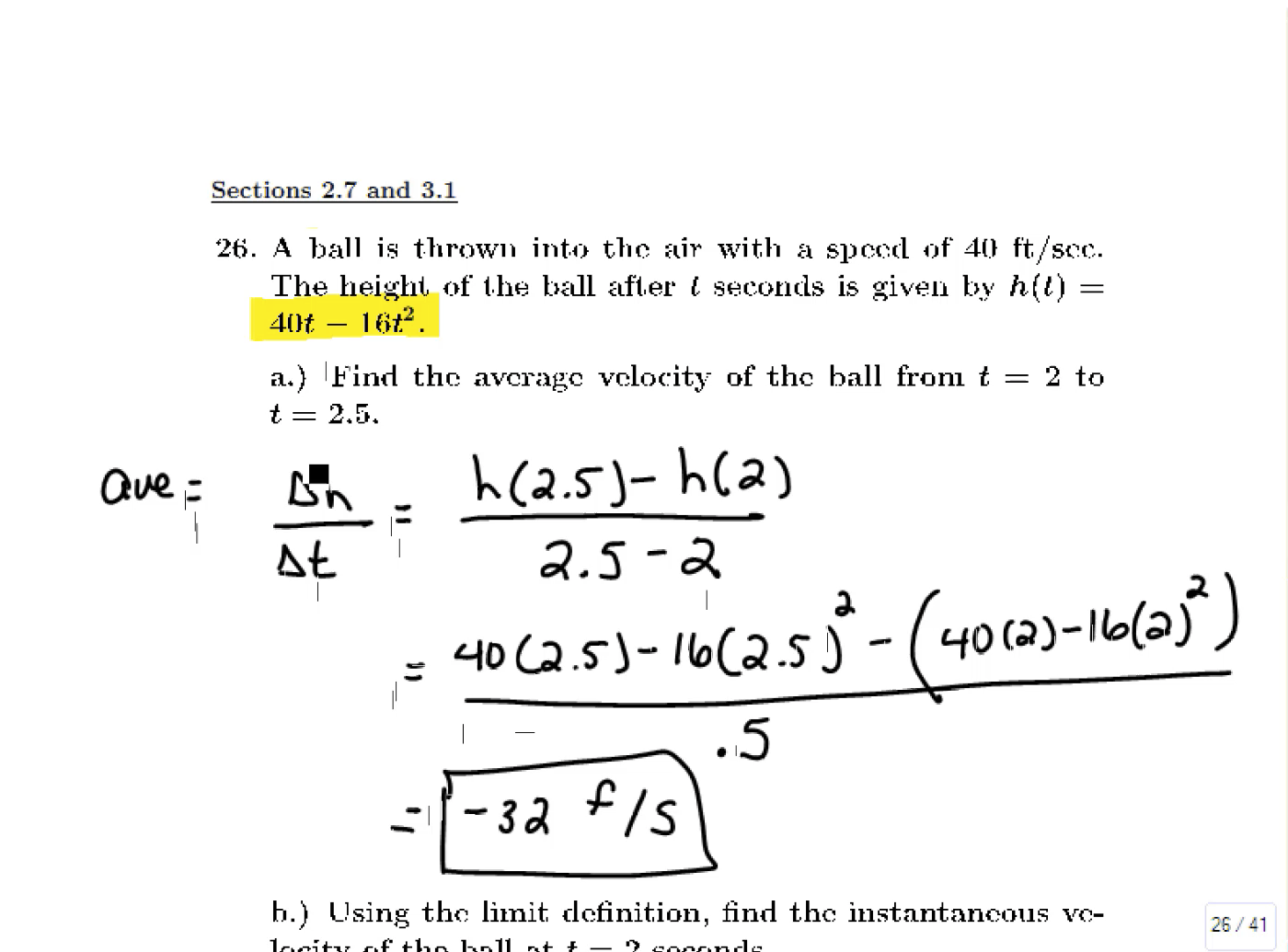
Review of the limit definition of a derivative and calculating the derivative
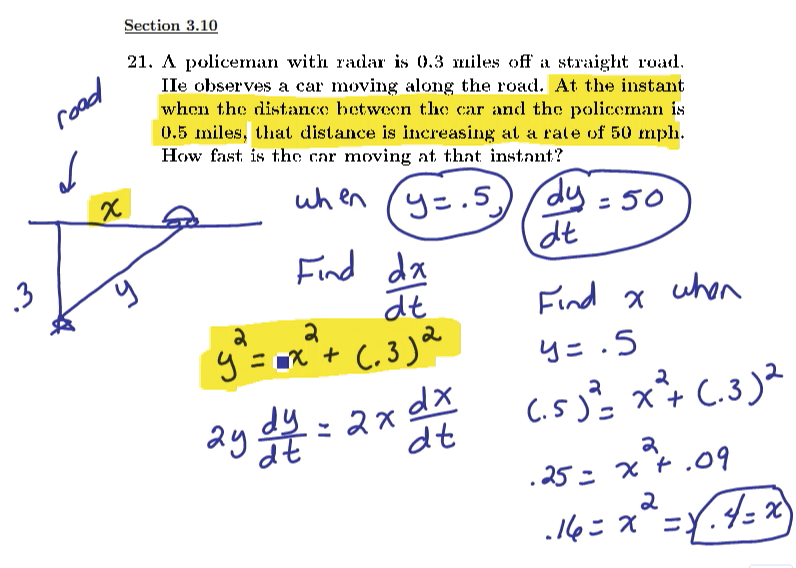
Related rates problems, differentials, linear and quadratic approximations

Review of the limit definition of a derivative and calculating the derivative
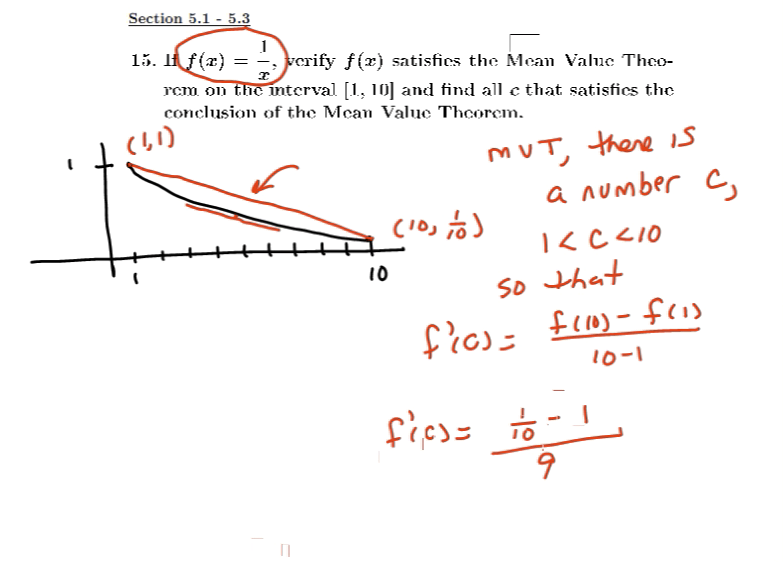
Mean Value Theorem and using derivatives to find the shape of curves
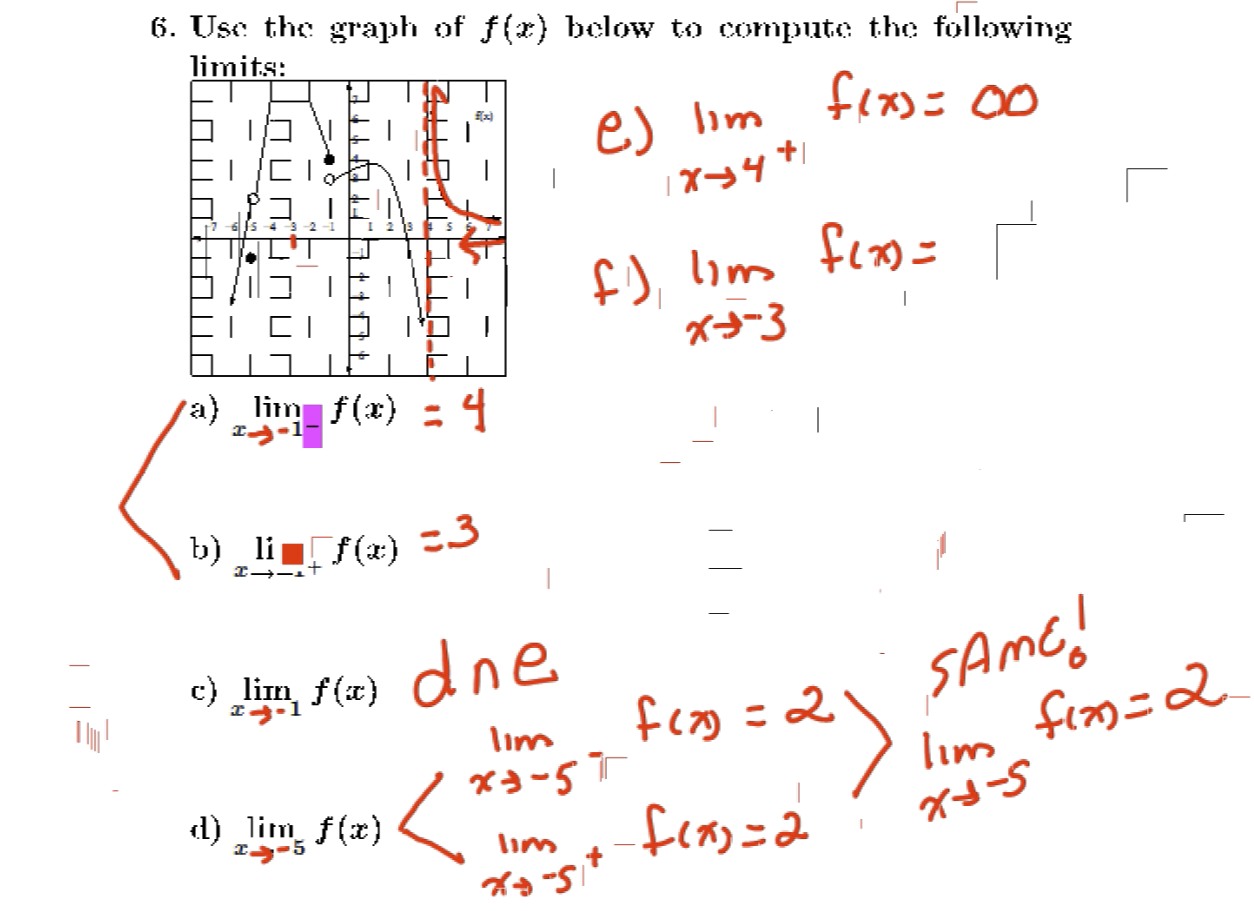
Evaluating limits of functions
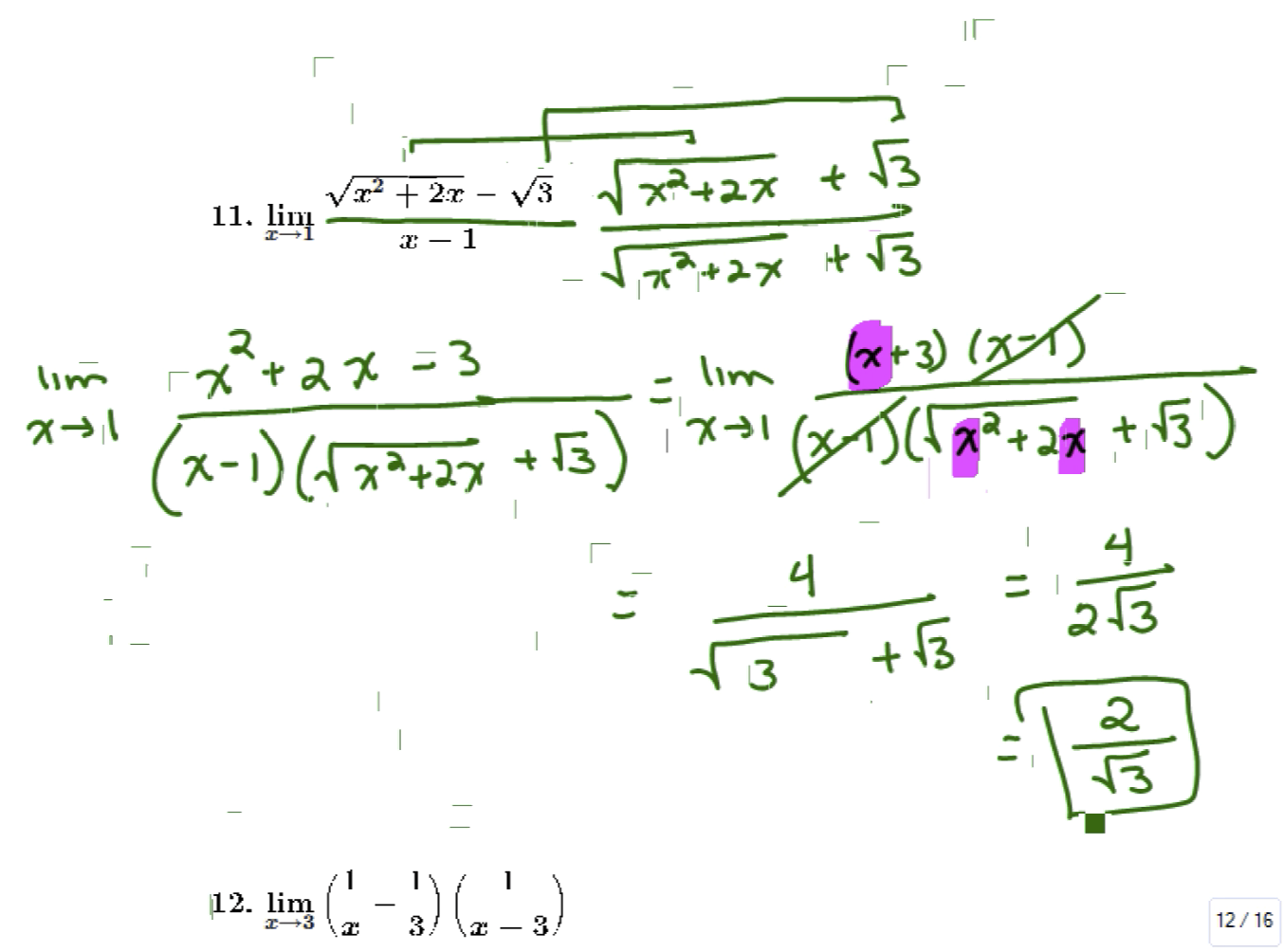
Evaluating Limits of Functions

Limits at infinity and asymptotes, along with physics applications
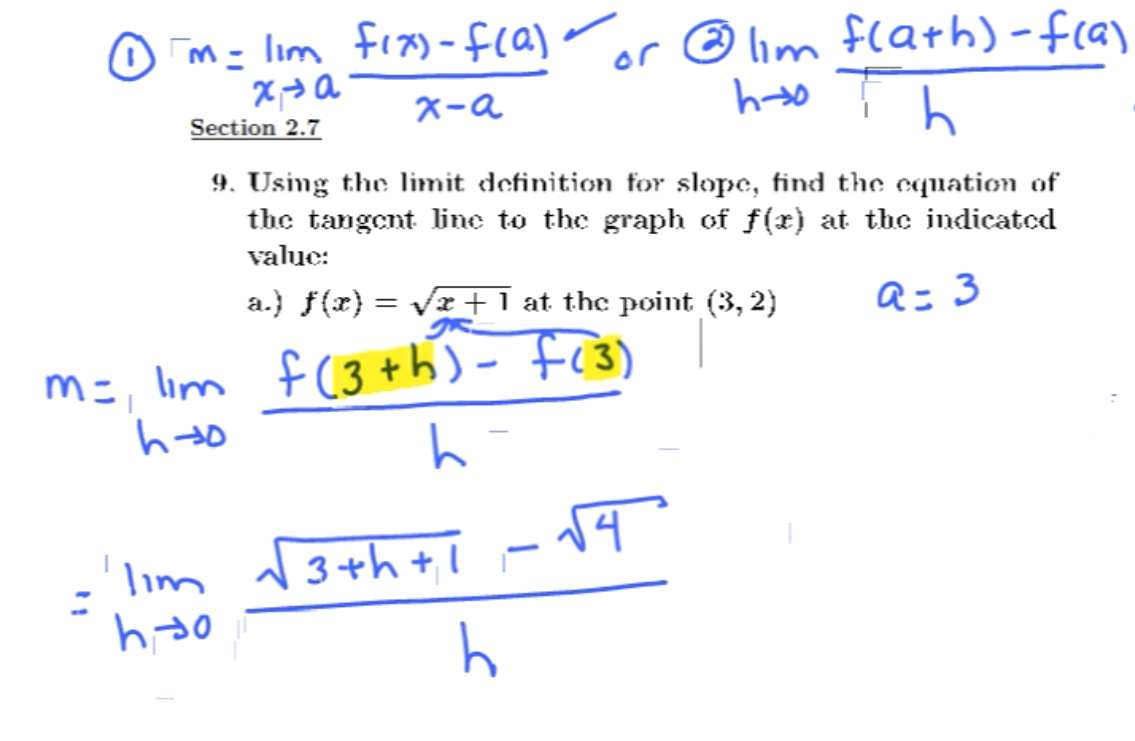
Limits at infinity, asymptotes, and tangent lines

Review of the limit definition of a derivative and calculating the derivative

Review of the limit definition of a derivative and calculating the derivative

Using derivatives to find properties of graphs
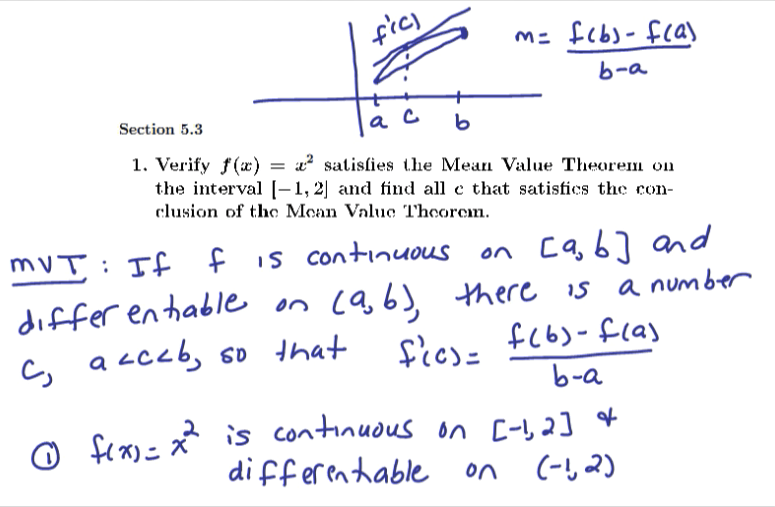
Mean Value Theorem and properties of a graph

Mean Value Theorem and properties of a graph
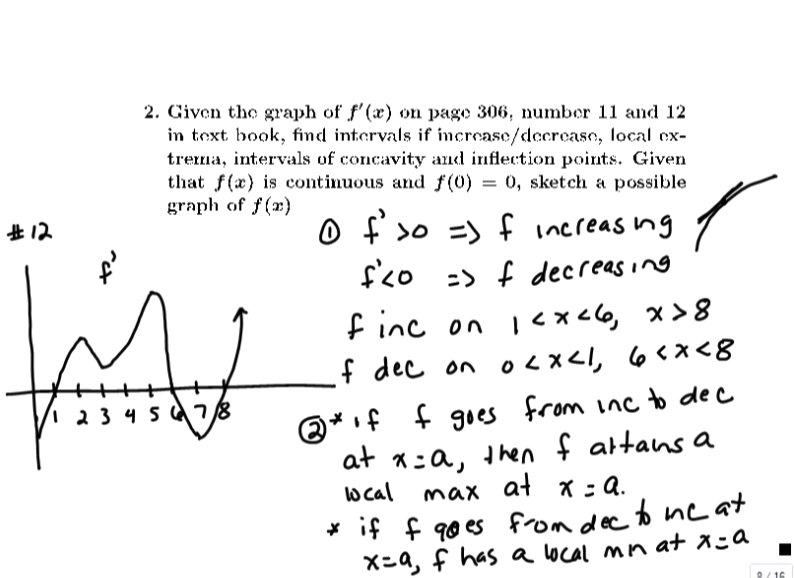
Using derivatives to find properties of graphs
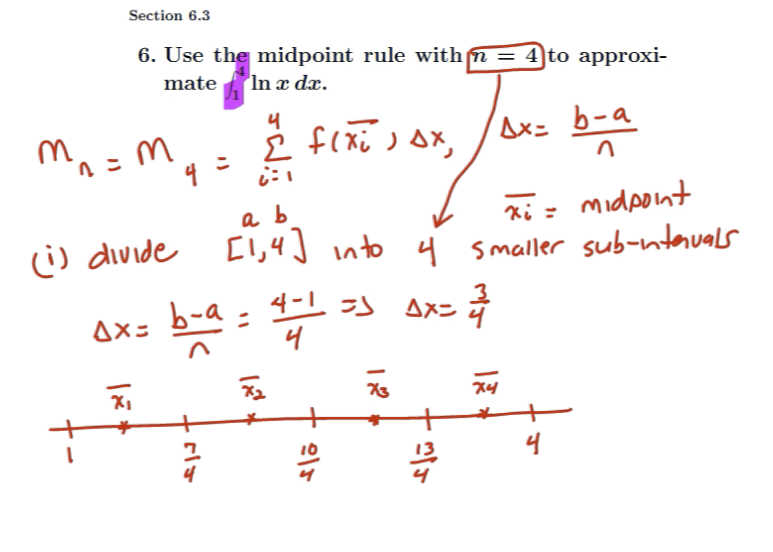
Using Reimann sums and the Fundamental Theorem of Calculus
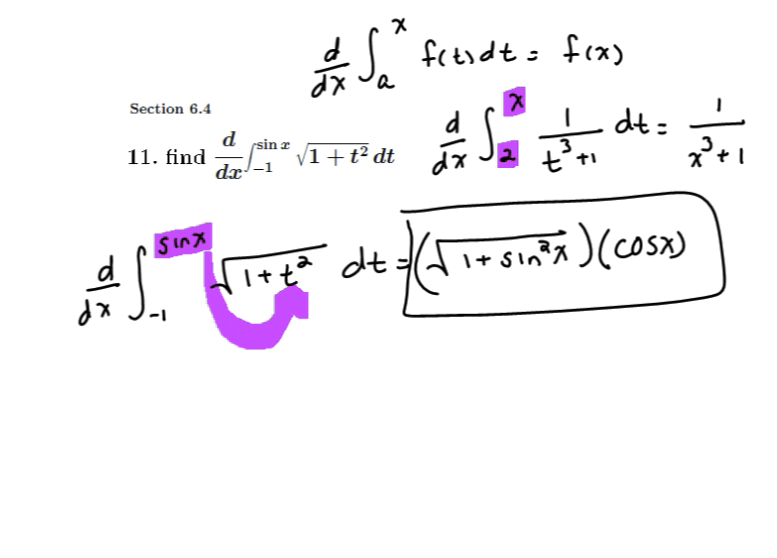
Using Riemann sums and the Fundamental Theorem of Calculus

Finding the vertical and horizontal asymptotes for a rational function

Using the Quotient Rule to differentiate a rational function

Review of limits, continuity, and the Intermediate Value Theorem

Review of the limit definition of a derivative and calculating the derivative

Related rates problems, differentials, linear and quadratic approximations

Review of the limit definition of a derivative and calculating the derivative

Mean Value Theorem and using derivatives to find the shape of curves

Evaluating limits of functions

Evaluating limits of functions and using the Squeeze Theorem

Proving a piecewise function and a polynomial are continuous

Limits at infinity and asymptotes, along with physics applications

Limits at infinity, asymptotes, and tangent lines

Review of the limit definition of a derivative and calculating the derivative

Review of the limit definition of a derivative and calculating the derivative

Using derivatives to find properties of graphs

Mean Value Theorem and properties of a graph

Mean Value Theorem and properties of a graph

Using derivatives to find properties of graphs

Using Reimann sums and the Fundamental Theorem of Calculus

Using Reimann sums and the Fundamental Theorem of Calculus

Finding the limit of a three-dimensional vector function

Creating symbolic expression in Python and then factoring, expanding, and simplifying the expressions

Finding the domain of a function with a square root in the denominator

Finding the domain of several rational functions

Finding the domain of a rational function

Explaining how to find any holes and vertical asymptotes of a rational function
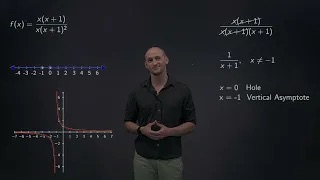
Finding the domain of a rational function using factoring

Finding the domain of a function with a denominator, square root, and logarithm

Discussing properties of linear functions

Discussing the types of linear graphs including horizontal and vertical lines and their properties

Solving quadratic equations with the difference of two squares formula
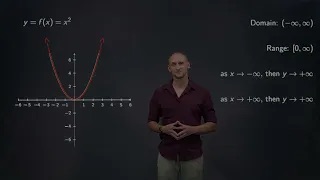
Explaining the graph and properties for the parent function of quadratics

Discussing properties of quadratic functions including the vertex, domain, range, and end behavior
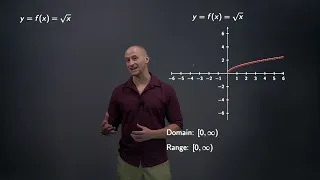
Explaining the general properties of even and odd root functions

Properties of the exponential function with base e

How to simplify rational expressions

Adding and subtracting rational expressions by finding a common denominator

Explaining how to multiply and divide rational expressions

Performing algebraic operations with rational expressions and simplifying the answer

Solving equations that contain rational expressions

Solving an equation that contains rational expressions

Solving an equation that contains rational expressions

Determining if functions are polynomials and finding their properties

Finding the domain of a piecewise function and evaluating it

Finding the properties of an exponential function including domain, range, end behavior, and intercepts

Finding the properties of an exponential function including domain, range, end behavior, and intercepts

Finding the properties of an exponential function including domain, range, end behavior, and intercepts
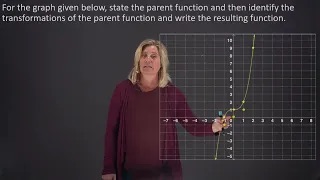
Identifying the parent function and transformations for a given graph

Finding the domain, range, end behavior, and intercepts for a logarithmic function

Adding and simplifying rational functions

Subtracting rational functions and then simplifying the result

Multiplying two rational functions and simplifying the result

Dividing rational functions and simplifying the result

Dividing and subtraction rational functions and simplifying the result

Computing and simplifying the difference quotient for a rational function

Finding the difference quotient for a quadratic function

Finding the limit of a rational function algebraically

Finding the limit of a rational function algebraically

Solving a limit approaching negative infinity for a rational function

Solving the limits approaching infinity and negative infinity of a rational function

Solving the limits approaching infinity and negative infinity of a rational function

Finding the holes and vertical asymptotes of a rational function

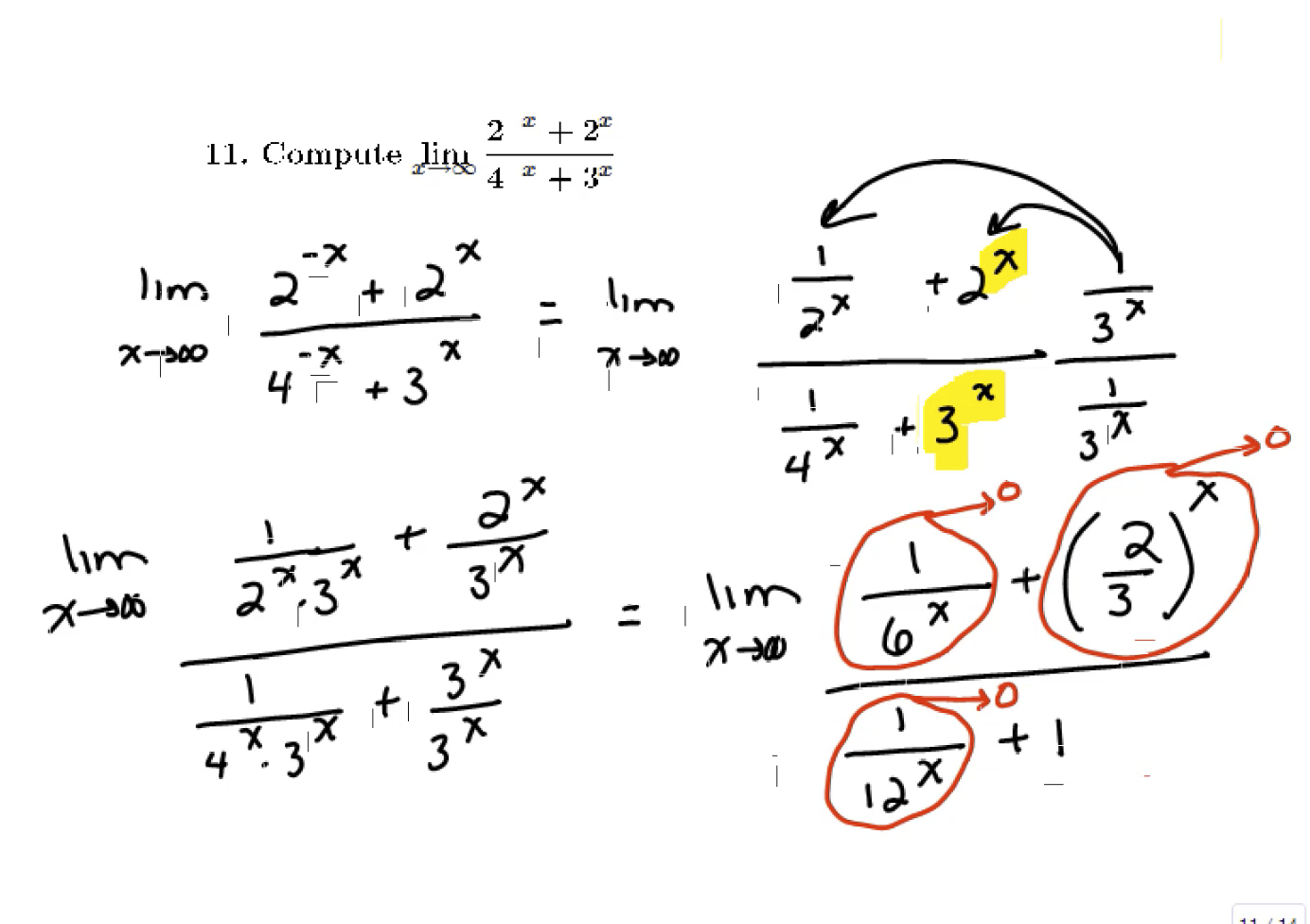
Finding the horizontal asymptotes of a function that is a fraction with exponentials in the numerator and denominator

Finding the exact value of the instantaneous rate of change

Using the limit definition to find the derivative of a polynomial

Using the limit definition to find the derivative of a rational function

Finding the derivative of a rational function by simplifying and using the power rule

Finding the derivative of a rational function using the Quotient Rule

Determining the intervals where a function is increasing and decreasing

Using the quotient rule twice to find the second derivative of a rational function

Finding the absolute maximum and minimum of a rational function on two closed intervals

Using the Intermediate Value Theorem and Bisection Method to approximate the solution of an equation

Calculating the derivative of a function with exponentials using the chain and product rule

Factoring a polynomial

Factoring a quadratic

Calculating the difference quotient for a rational function

Calculating the difference quotient for a rational function

Solving a polynomial equation using factoring by grouping

Finding the domains of expressions
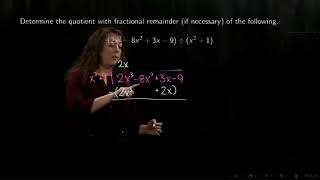
Calculating polynomial long division

Solving a nonlinear inequality
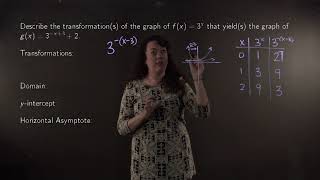
Determining properties of an exponential function

Determining properties of an exponential function
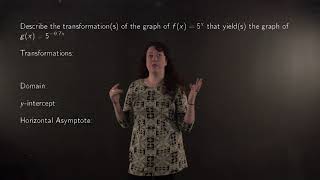
Determining properties of an exponential function
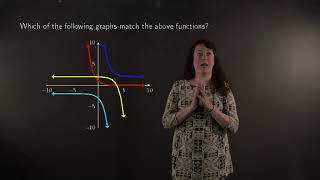
Identifying the graph of an exponential function

Determining properties of an exponential function

Cartesian equations and parametric equations of curves
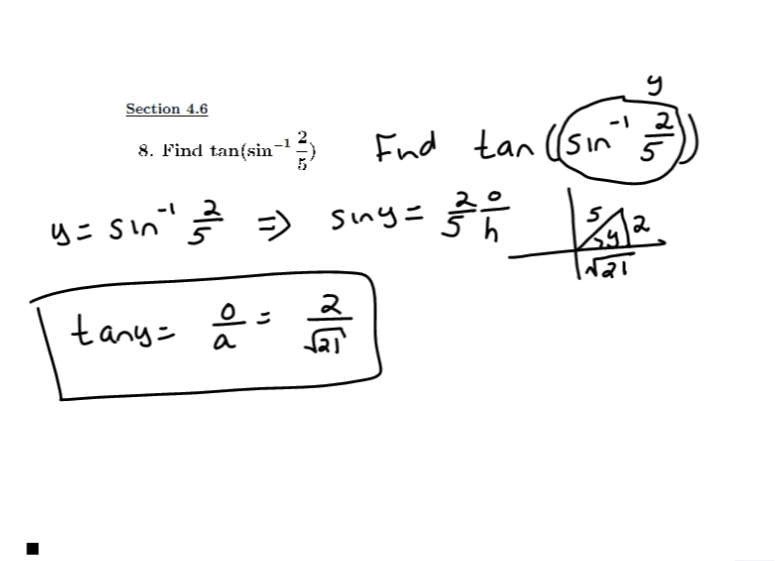
Review of limits and derivatives of inverse trigonometric functions

Reviewing the chain rule and the derivatives and limits of trigonometric functions
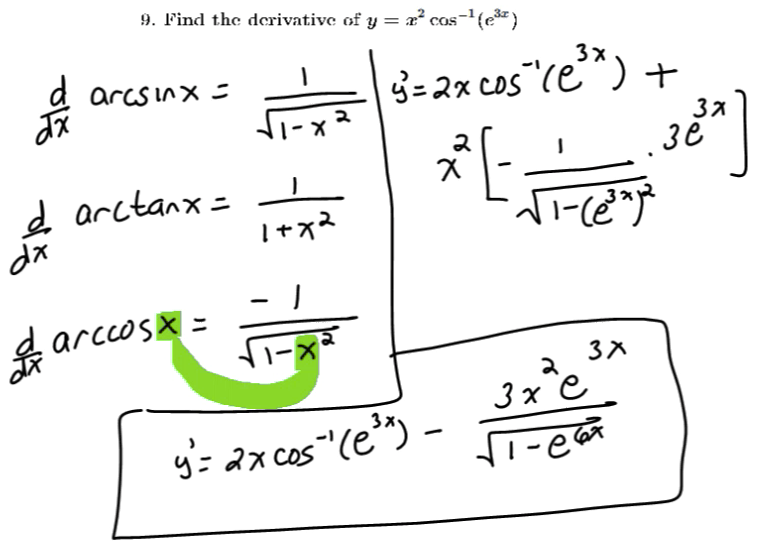
Review of limits and derivatives of inverse trigonometric functions

Antiderivatives and physics applications
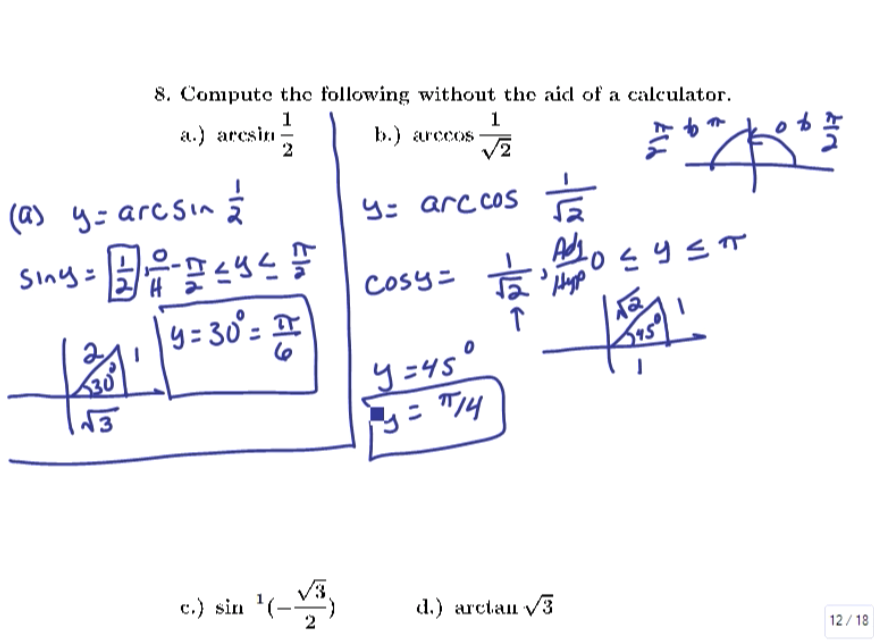
Properties and derivatives of inverse trigonometric functions

Continuity of Functions and the Intermediate Value Theorem
Approximation and Newton's Method, and limits and derivatives of exponential functions

Differentials, linear approximations and quadratic approximations

Derivatives of trigonometric functions and using the Chain Rule
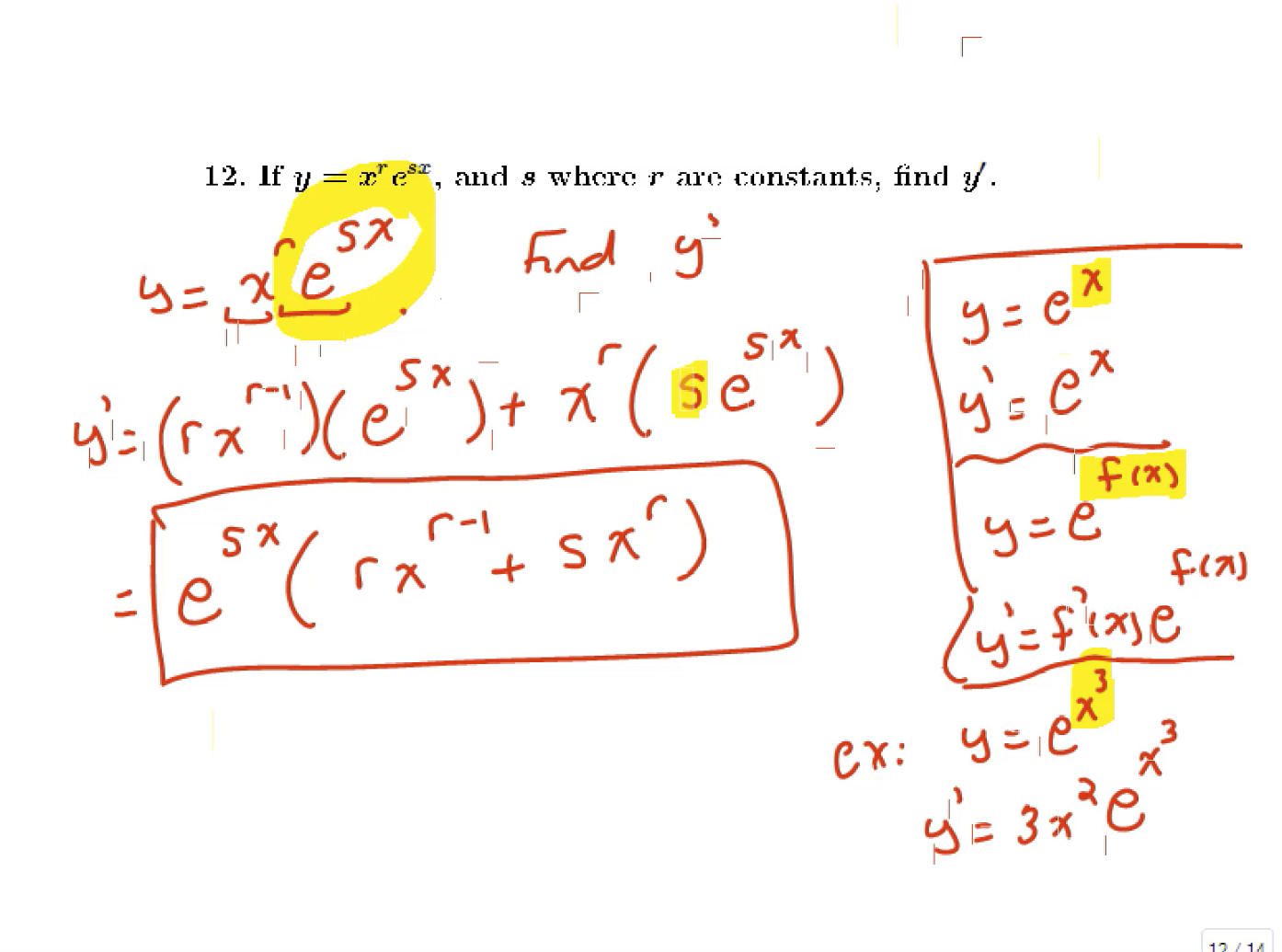
Approximation and Newton's Method, and limits and derivatives of exponential functions

Derivatives of trigonometric functions and using the Chain Rule

Properties and derivatives of inverse trigonometric functions
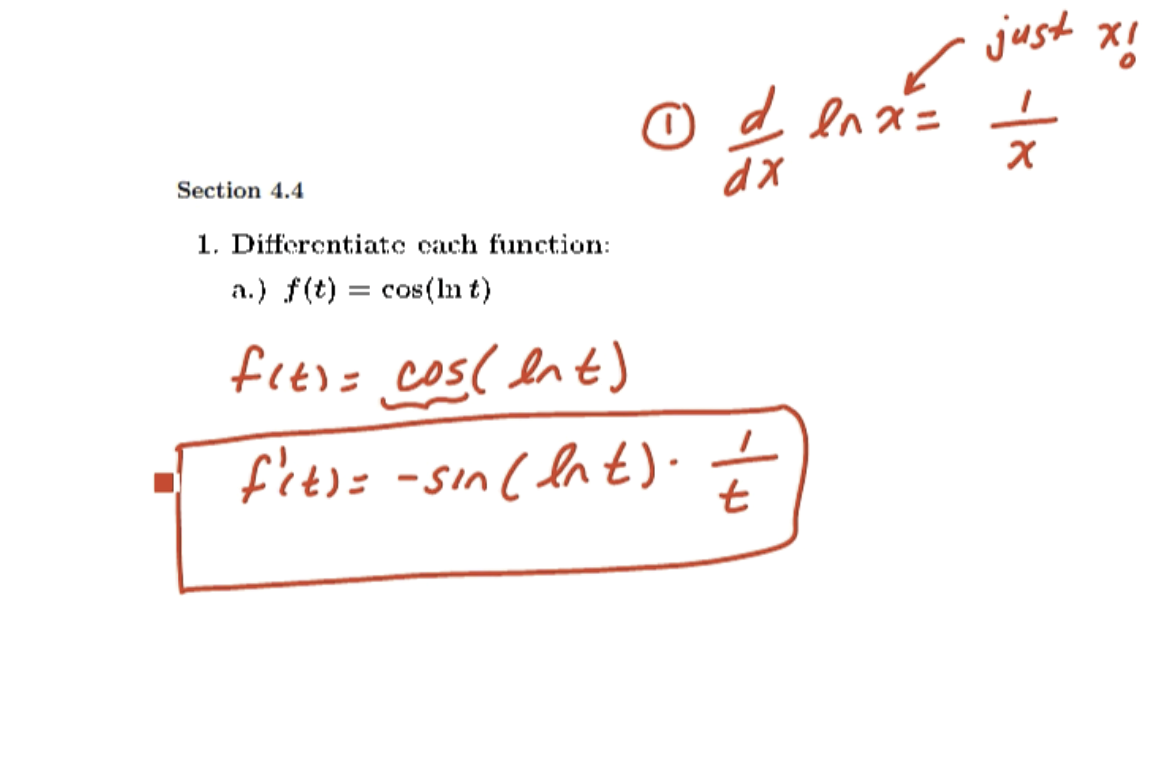
Derivatives of exponential and logarithmic functions and the exponential model

Derivatives of exponential and logarithmic functions and the exponential model

Riemann sums, including approximating areas under curves.
Examples of integration by substitution

Using the Product Rule to differentiate a function

Using the Product and Quotient Rules to differentiate a function

Using the Product Rule to find a first and second derivative

Differentiating functions using the Power Rule

Differentiating a function using the Power Rule

Differentiating a function with an exponential function and using the Power Rule

Using the Product Rule to differentiate a function

Using the Chain Rule to differentiate functions

Evaluating a limit using L'Hospital's Rule

Using the first and second derivatives to sketch the graph of a function

Finding the antiderivative of a polynomial

Finding the antiderivative of a polynomial

Finding a function from its second derivative using antidifferentiation

Antidifferentiating to find a function from its derivative

Antidifferentiating twice to find a function from its second derivative

Using antidifferentiation to find the height of a cliff from the impact speed of a dropped stone
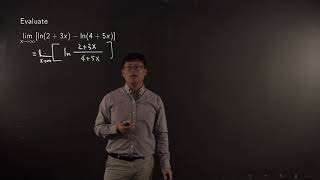
Evaluating a limit using logarithm properties

Evaluating a limit with an indeterminate difference using a common denominator

Finding the antiderivative of a polynomial

Finding the antiderivative of a rational function

Antidifferentiating twice to find a function from its second d

Antidifferentiating twice to find a function from its second d

Evaluating a a limit from the left for a rational function

Evaluating the limit of a rational function by factoring and cancelling

Using L'Hospital's Rule to evaluate a limit representing a derivative at a point

Evaluating the limit at infinity of a rational function

Evaluating a definite integral containing a polynomial

Evaluating a definite integral by first simplifying the integrand

Using u-substitution to evaluate an indefinite integral

Using u-substitution to evaluate an indefinite integral

Review of work and average value

Reviewing u-Substitution
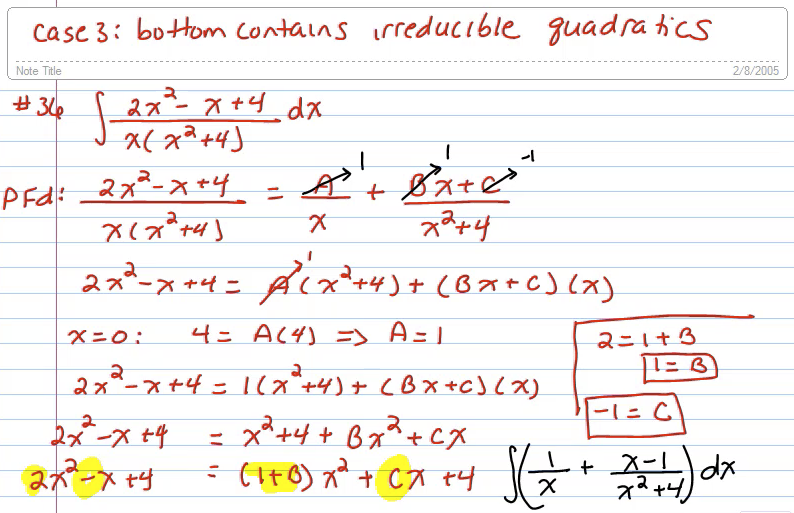
Review of partial fractions

Review of sequences and finding the sum of a series

Review of partial fractions

Integrating a rational function using u-substitution

Integrating a rational function using u-substitution

Using u-substitution to integrate a rational function

Explaining all four cases of partial fraction decomposition

Using partial fraction decomposition to rewrite and then integrate a rational function
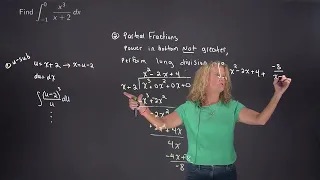
Using partial fraction decomposition to rewrite and then integrate a rational function

Using partial fraction decomposition to rewrite and then integrate a rational function

Using partial fraction decomposition to rewrite and then integrate a rational function

Using partial fraction decomposition to rewrite and then integrate a rational function

Proving a certain parameterization gives the unit circle and showing vector equations of lines are parallel

Cartesian equations and parametric equations of curves
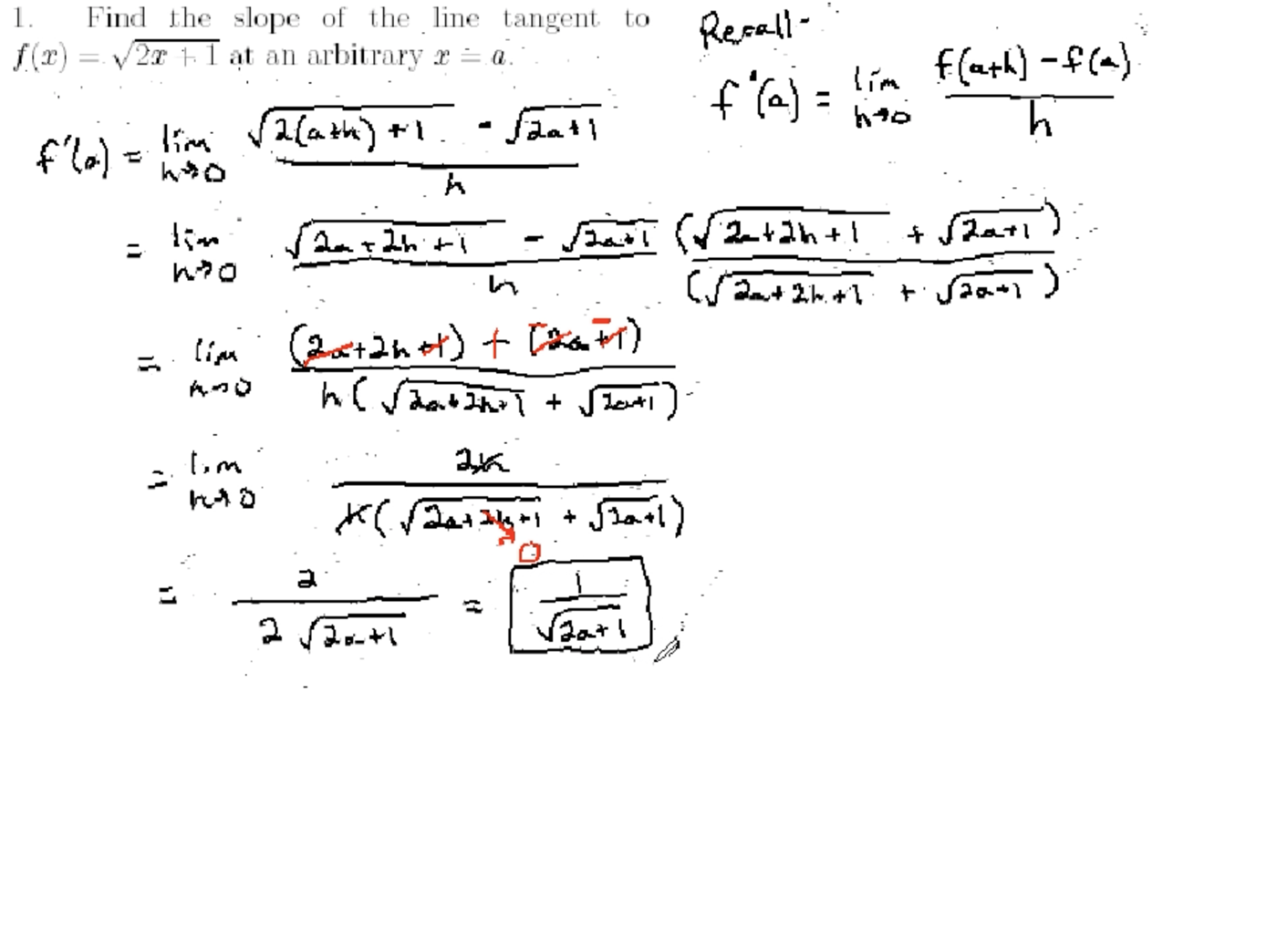
Using the limit definition to find derivatives of functions and vector functions

Calculating slopes of tangent lines to parametric curves

Review of limits and derivatives of inverse trigonometric functions

Reviewing the chain rule and the derivatives and limits of trigonometric functions

Review of limits and derivatives of inverse trigonometric functions

Antiderivatives and physics applications

Properties and derivatives of inverse trigonometric functions

Proving a product of continuous functions is continuous and using the Intermediate Value Theorem

Continuity of Functions and the Intermediate Value Theorem

Approximation and Newton's Method, and limits and derivatives of exponential functions

Using the limit definition to find derivatives of functions and vector functions

Using the limit definition to find derivatives of functions and vector functions

Differentials, linear approximations, and quadratic approximations

Finding a formula for a higher order derivative and a formula for the second derivative of a product

Derivatives of trigonometric functions and using the Chain Rule

Using the Chain Rule to prove facts about derivatives
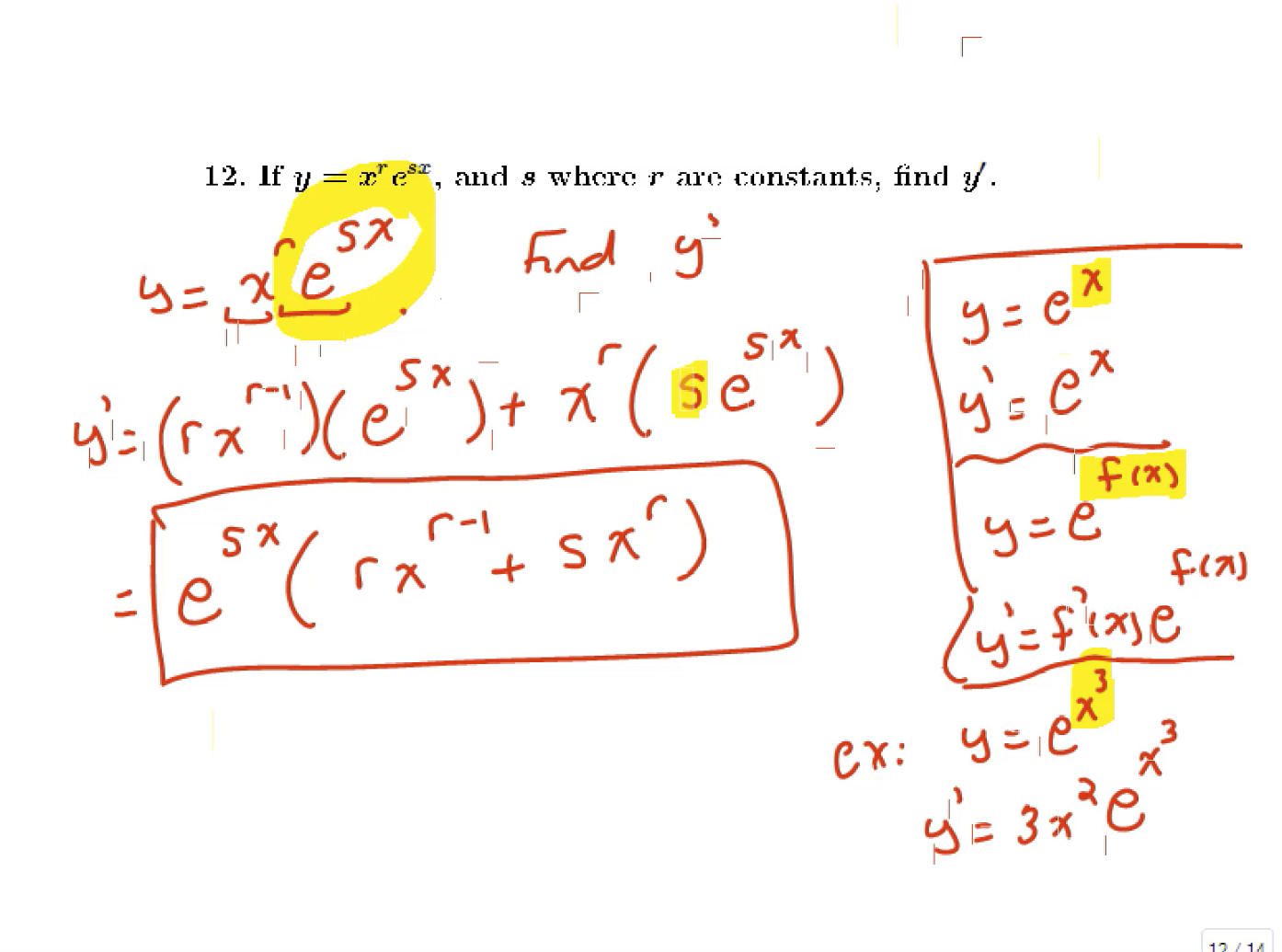
Approximation and Newton's Method, and limits and derivatives of exponential functions

Derivatives of trigonometric functions and using the Chain Rule

Finding a formula for a higher order derivative and a formula for the second derivative of a product
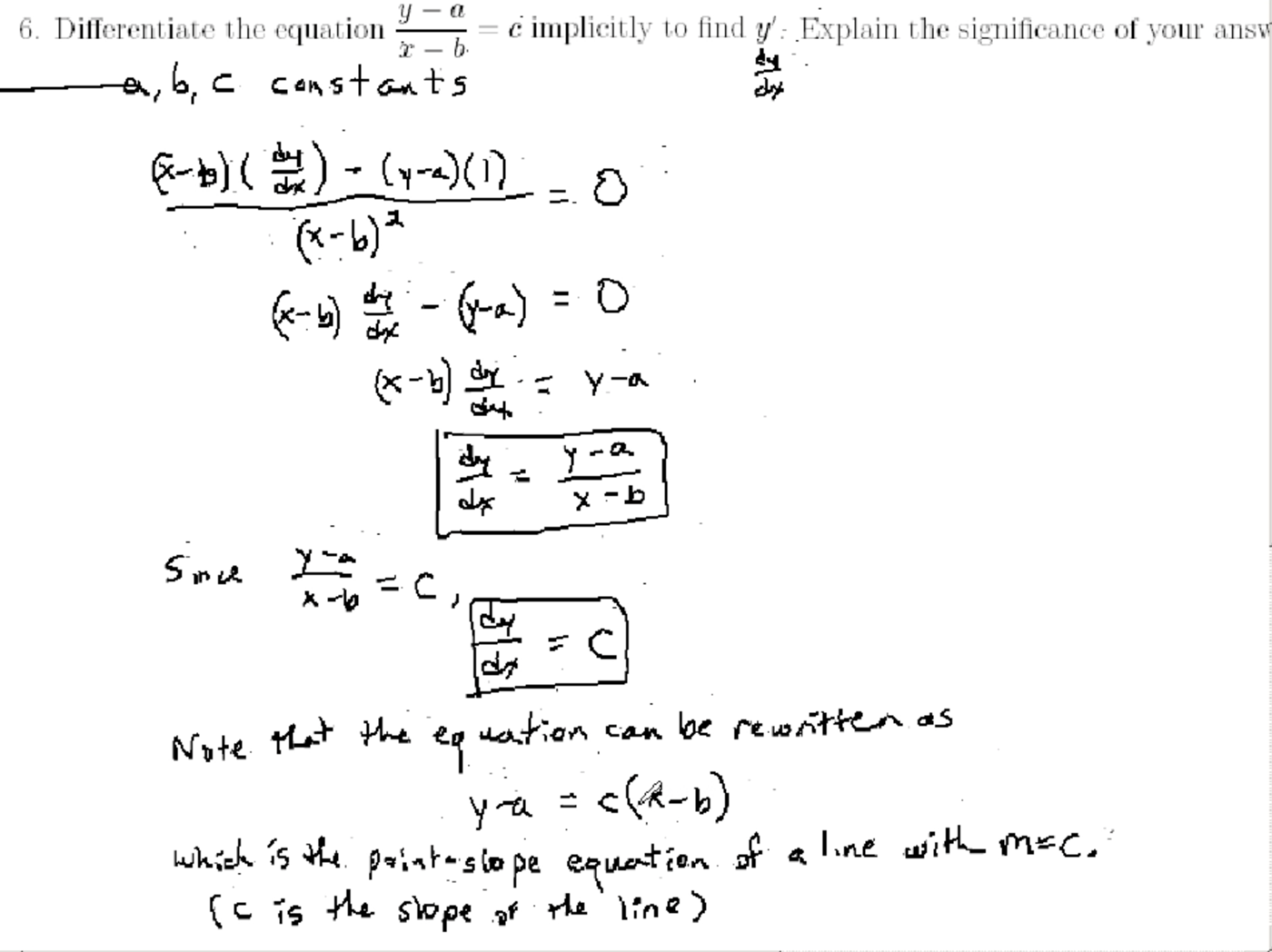
Using implicit differentiation to solve a problem and to prove the power rule for rational exponents

Properties and derivatives of inverse trigonometric functions
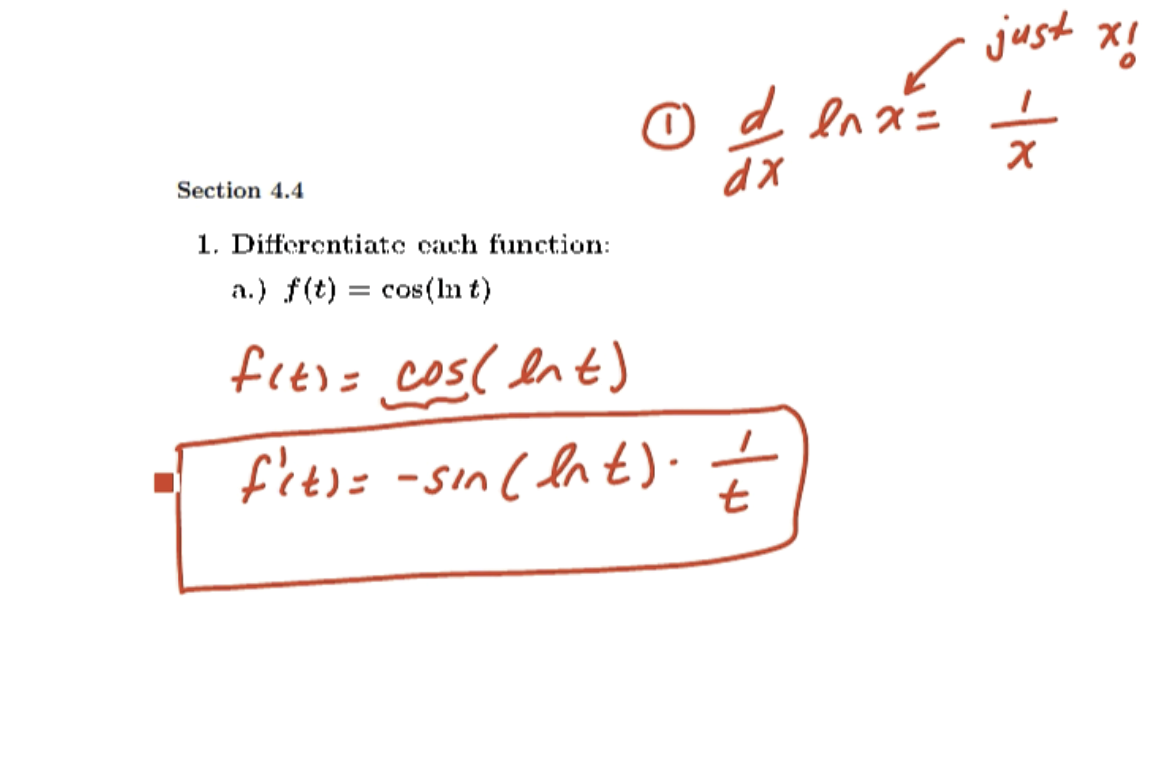
Derivatives of exponential and logarithmic functions and the exponential model

Using the Mean Value Theorem to prove facts about functions

Riemann sums, including approximating areas under curves.
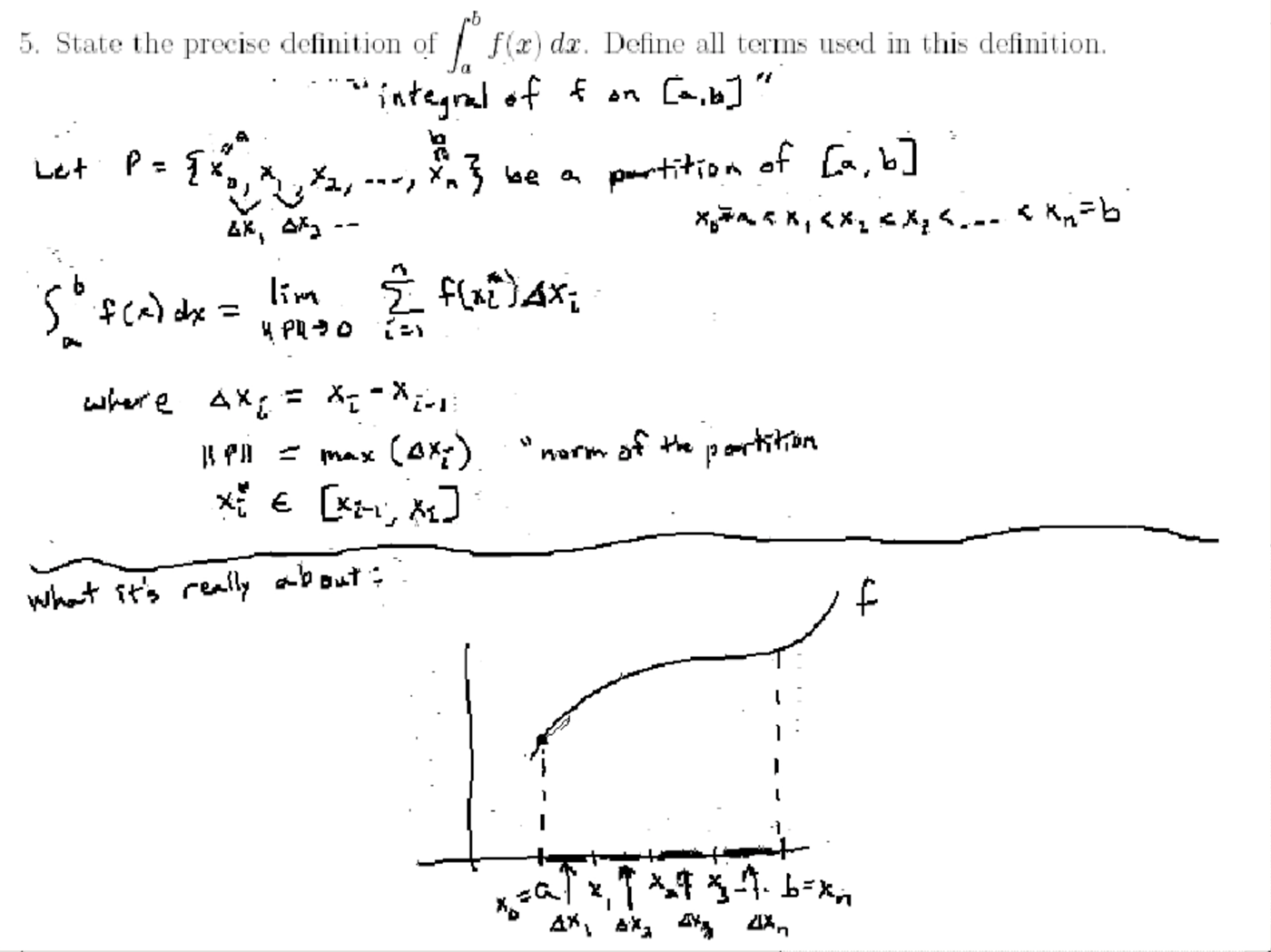
The definition and properties of definite integrals

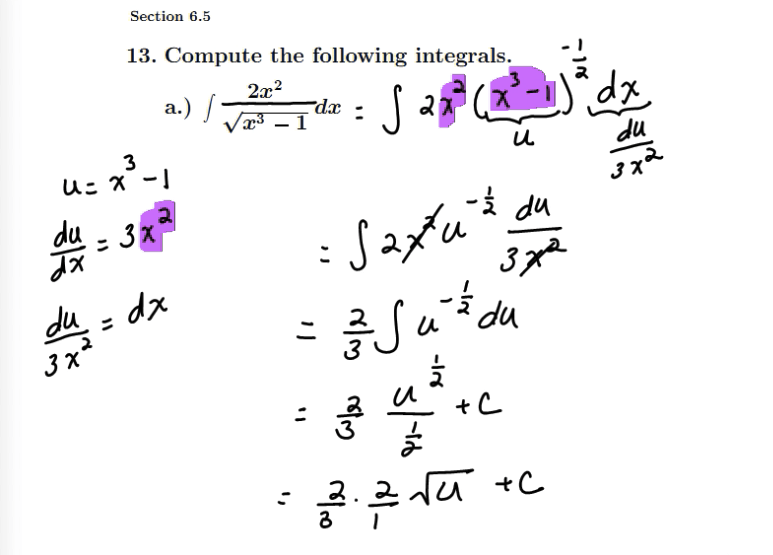
Examples of integration by substitution

Review of work and average value

Reviewing u-Substitution

Review of partial fractions

Review of sequences and finding the sum of a series

Review of partial fractions
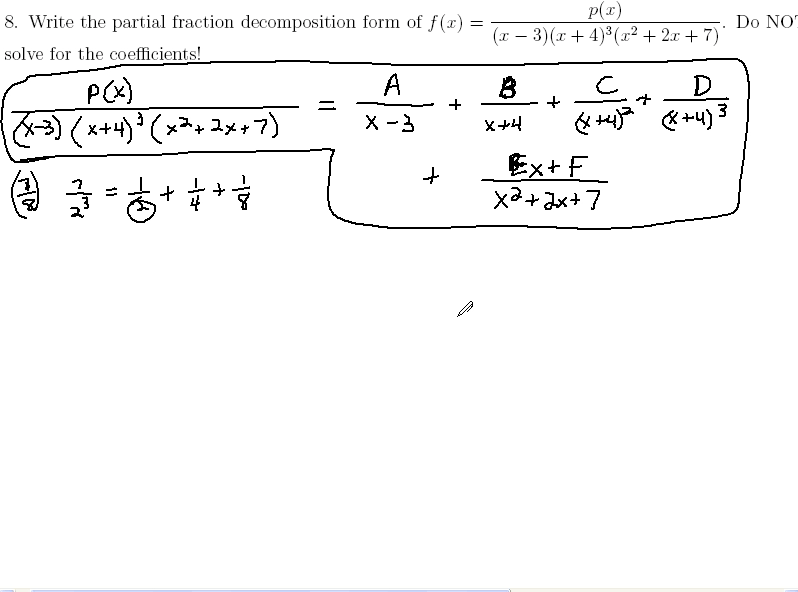
Finding a partial fraction decomposition and integrating using partial fractions

Explaining all four cases of partial fraction decomposition

Determining the domain of a three-dimensional vector function

Finding a vector equation for the tangent line to a three-dimensional vector function

Finding the angle of intersection for two three-dimensional vector functions

Graphing a piecewise function using Python

Finding the domain of a function with a natural logarithm and denominator

Finding the domain of a function with a root and a logarithm in the denominator
Finding the domain of a logarithmic function

Finding the domain of even and odd roots
Finding the domain of functions with even and odd roots

Explaining domain restrictions for denominators, even roots, and logarithms

Explaining the main three forms for linear equations

Discussing how to write the equation of a line through two given points

Writing a linear equation of profit from a business word problem

Solving a linear equation algebraically and showing how this relates to the graph
Solving a linear equation algebraically

Solving a linear equation algebraically and showing how this relates to the graph

Explaining the format and possible solutions for a system of two linear equations

Solving a system of linear equations with two variables

Solving a system of two linear equations with two variables using the elimination method

Solving a system of two linear equations using a TI-84 calculator

Solving a system of two linear equations using a TI-84 calculator

Solving a system of two linear equations using a TI-84 calculator

Explaining the standard form for a quadratic equation and the possible number of solutions

Solving quadratic equations by factoring

Solving a quadratic equation by factoring

Solving a quadratic equation by factoring

Solving quadratic equations with the quadratic formula and discussing the number of possible solutions

Explaining when a quadratic equation does not have a real solution

How to rewrite radical terms as power terms

Explaining how to solve radical equations and then solving example problems
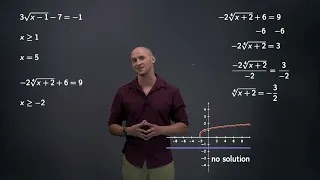
Solving equations with even roots and checking the solutions

Solving equations with odd radicals

Solving equations with multiple radicals

Simplifying power and exponential expressions using properties of exponents

Simplifying power and exponential expressions using properties of exponents

Simplifying power and exponential expressions using properties of exponents

Simplifying an expression with both power and exponential functions using properties of exponents

Explaining how to solve equations with power and exponential terms
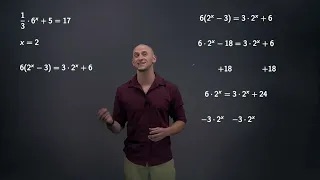
Solving equations with exponential terms

Solving equations with base e exponential terms
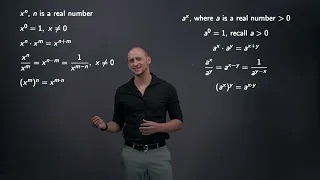
Properties of exponents applied to power and exponential expressions

Properties of multiplying and simplifying power and exponential expressions

Properties of exponents applied to power and exponential expressions

Expanding logarithmic expressions using logarithm rules

Condensing a logarithmic expression into a single logarithm

Explaining how to solve logarithmic equations

Solving logarithmic equations with a single logarithm term

Solving logarithmic equations with multiple logarithm terms

Solving logarithmic equations with multiple logarithm terms on both sides

Explaining the terminology and rules of logarithms

Explaining basic algebraic operations for fractions

Explaining all four cases of partial fraction decomposition

Explaining the format and possible solutions for a system of two linear equations

Determining whether polynomials are linearly independent

Showing that a set of polynomials forms a basis for all polynomials with degree two or less

Solving a rational equation and checking the solutions

Finding the domain, intercepts, holes, and vertical asymptotes for a rational function

Compute and simplify the difference quotient for a rational function